Question 10623 – GATE 1987
June 2, 2024Question 17270 – NTA UGC NET JUNE 2023 Paper-1
June 2, 2024Question 10586 – GATE 1989
Which of the following graphs is/are planner?
Correct Answer: A
Question 13 Explanation:
(i) G1 is K33 which is planar graph with the minimum number of edges.
→ Let us assume K33is a planar graph. Then it satisfy the useful corollary. As there is no triangle in K33.
Let G be a connected planar graph with n vertices and m edges, and no triangles. Then m≤2n-4.
Where m=9, n=6
⇒ 9 ≤ 12 – 4
⇒ 9 ≤ 8, which is to be false, then K33 is non-planar graph.
(ii) G2 is a planar graph. Because it can be redrawn like as below.

(iii) Let us assume G3 be a planar graph then it also be satisfy useful corollary.
Where m=9, n=6
then 9 ≤ 12-4
9 ≤ 8 is False
So, G3 is non-planar graph.
Answer: Only G2 is planar graph.
→ Let us assume K33is a planar graph. Then it satisfy the useful corollary. As there is no triangle in K33.
Let G be a connected planar graph with n vertices and m edges, and no triangles. Then m≤2n-4.
Where m=9, n=6
⇒ 9 ≤ 12 – 4
⇒ 9 ≤ 8, which is to be false, then K33 is non-planar graph.
(ii) G2 is a planar graph. Because it can be redrawn like as below.
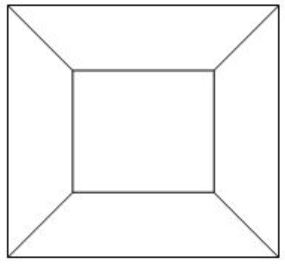
(iii) Let us assume G3 be a planar graph then it also be satisfy useful corollary.
Where m=9, n=6
then 9 ≤ 12-4
9 ≤ 8 is False
So, G3 is non-planar graph.
Answer: Only G2 is planar graph.
Theory Explanation is given below.
Subscribe
Login
0 Comments

