Question 8815 – Engineering-Mathematics
Let A be the 2×2 matrix with elements a11 = a12 = a21 = +1 and a22 = -1. Then the eigenvalues of the matrix A19 are
Correct Answer: D
Question 142 Explanation:
a11 = a12 = a21 = 1, a22 = -1
The 2×2 matrix =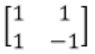
Cayley Hamilton theorem:
If matrix A has ‘λ’ as eigen value, An has eigen value as λn.
Eigen value of
|A-λI| = 0

-(1-λ)(1+λ)-1 = 0
-(1-λ2 )-1 = 0
-1 = 1-λ2
λ2 = 2
λ = ±√2
A19 has (√2)19 = 29×√2 (or) (-√2)19 = -512√2
= 512√2
The 2×2 matrix =
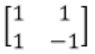
Cayley Hamilton theorem:
If matrix A has ‘λ’ as eigen value, An has eigen value as λn.
Eigen value of

|A-λI| = 0

-(1-λ)(1+λ)-1 = 0
-(1-λ2 )-1 = 0
-1 = 1-λ2
λ2 = 2
λ = ±√2
A19 has (√2)19 = 29×√2 (or) (-√2)19 = -512√2
= 512√2
1024 and -1024
1024√2 and -1024√2
4√2 and -4√2
512√2 and -512√2
