Prepositional-Logic
Question 1 |
The proposition p ∧(~p ∨ q) is:
a tautology | |
logically equivalent to p ∧ q | |
logically equivalent to p ∨ q | |
a contradiction | |
none of the above |
Question 1 Explanation:
p ∧(~p ∨ q)
(p ∧ ~p) ∨ (p ∧ q)
F ∨ (p ∧ q)
(p ∧ q)
(p ∧ ~p) ∨ (p ∧ q)
F ∨ (p ∧ q)
(p ∧ q)
Question 2 |
Let p and q be propositions. Using only the truth table decide whether p ⇔ q does not imply p → q is true or false.
True | |
False |
Question 2 Explanation:
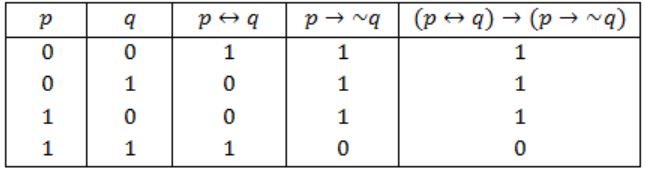
So, "imply" is False making "does not imply" True.
Question 3 |
A language with string manipulation facilities uses the following operations
head(s): first character of a string tail(s): all but the first character of a string concat(s1,s2):s1 s2 for the string acbc what will be the output of concat(head(s), head(tail(tail(s))))
ac | |
bc | |
ab | |
cc |
Question 3 Explanation:
concat (a, head (tail (tail (acbc))))
concat (a, head (tail (cbc)))
concat (a, head (bc))
concat (a, b)
ab
concat (a, head (tail (cbc)))
concat (a, head (bc))
concat (a, b)
ab
Question 4 |
If the proposition ¬p ⇒ ν is true, then the truth value of the proposition ¬p ∨ (p ⇒ q), where ¬ is negation, ‘∨’ is inclusive or and ⇒ is implication, is
true | |
multiple valued | |
false | |
cannot be determined |
Question 4 Explanation:
From the axiom ¬p → q, we can conclude that p ∨ q.
So, either p or q must be True.
Now,
¬p ∨ (p → q)
= ¬p ∨ (¬p ∨ q)
= ¬p ∨ q
Since nothing c an be said about the truth values of p, it implies that ¬p ∨ q can also be True or False. Hence, the value cannot be determined.
So, either p or q must be True.
Now,
¬p ∨ (p → q)
= ¬p ∨ (¬p ∨ q)
= ¬p ∨ q
Since nothing c an be said about the truth values of p, it implies that ¬p ∨ q can also be True or False. Hence, the value cannot be determined.
Question 5 |
Choose the correct alternatives (more than one may be correct) and write the corresponding letters only: If F1, F2 and F3 are propositional formulae such that F1 ∧ F2 → F3 and F1 ∧ F1 → ~F2 are both tautologies, then which of the following is true:
Both F1 and F2 are tautologies | |
The conjunction F1 ∧ F2 is not satisfiable | |
Neither is tautologous | |
Neither is satisfiable | |
None of the above |
Question 5 Explanation:
For the propositional formula A→B to be tautology the T→F condition should never arise.
So, in option (B) it is saying that F< sub>1 ∧ F2 is not satisfiable means F1 ∧ F2 is always false.
And False → anything is always true.
So, in option (B) it is saying that F< sub>1 ∧ F2 is not satisfiable means F1 ∧ F2 is always false.
And False → anything is always true.
Question 6 |
Which of the following predicate calculus statements is/are valid:
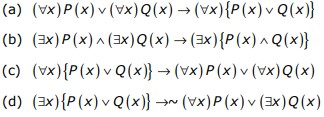
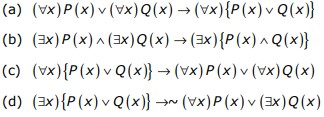
a | |
b | |
c | |
d |
Question 6 Explanation:
(A) Valid
(B) Invalid
(C) Invalid
(D) Invalid
(B) Invalid
(C) Invalid
(D) Invalid
There are 6 questions to complete.
