Digital-Logic-Design
October 15, 2023Digital-Logic-Design
October 15, 2023Digital-Logic-Design
| Question 548 |
The decimal floating point number -40.1 represented using IEEE-754 32-bit representation and written in hexadecimal form is
| 0xC2206000 | |
| 0xC2006666 | |
| 0xC2006000 | |
| 0xC2206666 |
Question 548 Explanation:
1. Fraction part can be converted into binary form by multiplying it with 2 and taking non fractional part of the product. Take fractional part and multiply again as explained above.
0.1 x 2= 0.2 → 0
0.2 x 2= 0.4 → 0
0.4 x 2= 0.8 → 0
0.8 x 2= 1.6 → 1
0.6 x 2= 1.2 → 1
(0.1) 10 = (0.00011) 2
(40) 10 = (101000) 2
101000.00011
Normalize the number
1.0100000011 x 2 5
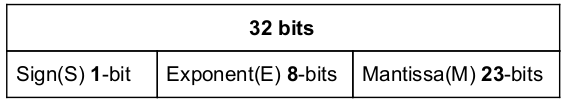
Biased exponent= 5+127= 132=(1000 0100) 2
Mantissa= 01000000110000000000000
Sign= 1

0.1 x 2= 0.2 → 0
0.2 x 2= 0.4 → 0
0.4 x 2= 0.8 → 0
0.8 x 2= 1.6 → 1
0.6 x 2= 1.2 → 1
(0.1) 10 = (0.00011) 2
(40) 10 = (101000) 2
101000.00011
Normalize the number
1.0100000011 x 2 5
Biased exponent= 5+127= 132=(1000 0100) 2
Mantissa= 01000000110000000000000
Sign= 1
Correct Answer: D
Question 548 Explanation:
1. Fraction part can be converted into binary form by multiplying it with 2 and taking non fractional part of the product. Take fractional part and multiply again as explained above.
0.1 x 2= 0.2 → 0
0.2 x 2= 0.4 → 0
0.4 x 2= 0.8 → 0
0.8 x 2= 1.6 → 1
0.6 x 2= 1.2 → 1
(0.1) 10 = (0.00011) 2
(40) 10 = (101000) 2
101000.00011
Normalize the number
1.0100000011 x 2 5
Biased exponent= 5+127= 132=(1000 0100) 2
Mantissa= 01000000110000000000000
Sign= 1

0.1 x 2= 0.2 → 0
0.2 x 2= 0.4 → 0
0.4 x 2= 0.8 → 0
0.8 x 2= 1.6 → 1
0.6 x 2= 1.2 → 1
(0.1) 10 = (0.00011) 2
(40) 10 = (101000) 2
101000.00011
Normalize the number
1.0100000011 x 2 5
Biased exponent= 5+127= 132=(1000 0100) 2
Mantissa= 01000000110000000000000
Sign= 1

Subscribe
Login
0 Comments
